In today’s lesson, we will show a simple method for proving the Consecutive Interior Angles Converse Theorem.
The Consecutive Interior Angles Theorem states that the consecutive interior angles on the same side of a transversal line intersecting two parallel lines are supplementary (That is, their sum adds up to 180).
Here we will prove its converse of that theorem. We will show that if the consecutive interior angles on the same side of a transversal line intersecting two lines are supplementary, then the two lines are parallel.
Problem
m∠5 + m∠4 = 180°, prove that AB||CD
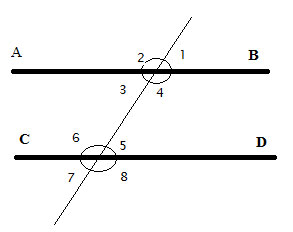
Strategy
This is a converse theorem. A converse of a theorem is a statement formed by interchanging what is given in a theorem and what is to be proved.
So it makes sense to start by looking at what we did in the original theorem. Then, we will try to replicate that.
In proving the original theorem, we relied on the fact that a linear pair of angles are supplementary. Let’s do that here, too: m∠1 + m∠4 = 180° as a linear pair, m∠5 + m∠4 = 180° is given, so m∠5=m∠1, and by the converse of the corresponding angles theorem, the lines are parallel.
And this is how you prove the Consecutive Interior Angles Converse Theorem!
Proof
(1) m∠5 + m∠4 = 180° //From the problem statement
(2) m∠1 + m∠4 = 180° // Linear pair of angles are supplementary
(3) m∠5=m∠1 // (1), (2) , transitive property of equality
(4) AB||CD // Converse of the corresponding angles theorem
