Area of a Parallelogram

In this lesson, we'll derive the formula for the area of a parallelogram in two ways.

First, we will use what we have already done when we found the area of a trapezoid - because we can think of a parallelogram as a kind of a trapezoid in which both "bases" happen to be parallel, not just one pair.

Then we will derive the formula directly, using partitioning into shapes whose area we already know how to compute - rectangles and triangles.

Problem

Find a formula for the area of a parallelogram using its height and side.

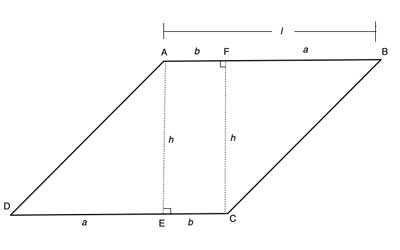
Strategy

Certainly, one of the basic strategies in solving geometry problems, which actually saves a lot of work, is to rely on things we have already done and proven previously and avoid having to redo everything from the starting point.

In this case, we note that when we derived the formula for the area of a trapezoid, the only property of the trapezoid we relied on was that it had one pair of opposite sides that were parallel to each other.

So, if we think of a parallelogram as a "special trapezoid", in which the other pair of opposite sides is also parallel, but ignore those sides (because they were not actually used in deriving the formula), we can use the trapezoid formula: A_Trapezoid=(short base+long base)·height/2.

But in the case of a parallelogram, the "short base" and"long base" are actually the same size, and equal to the side of the parallelogram, l. Substituting l into the above formula we get A_{Parallelogram}=(l+l)·height/2= 2·l·height/2=l·height.

So we have an easy solution. But suppose we hadn't already derived the trapezoid formula. We can derive the formula for the area of a parallelogram from scratch, following similar steps to what we did for a trapezoid: partition the parallelogram into simpler shapes that we already have the formula for (triangles and rectangles).

How to partition? The hint is given in the problem statement which says to use the height of the parallelogram, so let's construct that height, as in the sketch above. We will show that AFCE is a rectangle, and that the two triangles ΔAED and ΔCFB are congruent, find the areas of these three pieces, and add them up to get the area of the parallelogram.

Solution

(1) AE ⊥ DC //Construction
(2) CF ⊥ AB //Construction
(3) m∠AEC=90° //(1), definition of perpendicular lines
(4) AF||EC //Given, ABCD is a parallelogram
(5) m∠AEC=90° //Consecutive interior angles are supplementary
(6) m∠CFB=90° //(2), definition of perpendicular lines
(7) AE||FC //Converse of the Corresponding Angles Theorem
(8) AFCE is a rectangle //(4), (7) – definition of a parallelogram, (5), (6) definition of rectangle
(9) AF=EC=b //Opposing sides of rectangle are equal
(7) AE=CF=h //Opposing sides of rectangle are equal
(8) A_ABCD=A_AFCE+A_AED+A_BFC //Area of parallelogram is sum of area of its parts
(9) A_AFCE=b·h

Now let's calculate the area of the triangles:
(10) AE=CF=h //Opposing sides of rectangle are equal
(11) AD=BC //Given, Opposing sides of a parallelogram are equal
(12) m∠CFB=m∠AEC=90° //(1), (2) , Construction
(13) ∠CFB≅∠AEC //(12), defintion of congruent angles
(14) ΔAED ≅ ΔCFB //Hypotenuse-Leg
(15) A_AED=a·h/2
(16) A_BFC=a·h/2
(17) A_ABCD=b·h+a·h/2 + a·h/2=b·h+a·h= (a+b)·h = l·h