A line tangent to a circle is a line from a point outside the circle that touches the circle at exactly one point. At that point, the tangent line is perpendicular to the circle's radius and diameter, and this property is often used in high school geometry problems involving tangent lines.
Problem
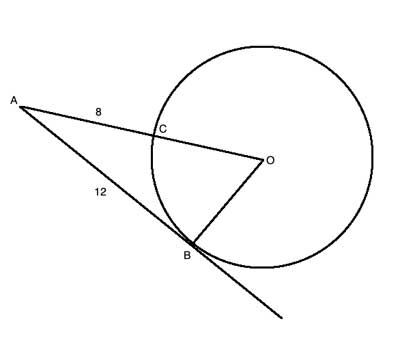
Line AB is tangent to circle O at point B, and the line segment AB measures 12 units. The line AO, from point A to the circle's center, intersects the circle at point C, and the line segment AC measures 8 units. Find the circle's radius.
Strategy
The problem features a tangent line, which is a hint to use the property of tangent lines to a circle: that line is perpendicular to the circle's radius at the point where it touches the circle.
So ∠OBA=90° and ΔOBA is a right triangle, whose long leg's length we know (AB=12). The circle's radius, which is what we need to find, is the short leg of the right triangle (OB).
Next, we note that the hypotenuse, AO, is made up of a segment whose length we know (AC=8) and another radius - OC. We can call the radius x, and solve for x using the Pythagorean Theorem.
Solution
(1) AB⊥OB //given, AB is tangent to circle O, a tangent line is perpendicular to the radius
(2) ∠OBA=90° //Definition of perpendicular line
(3) ΔOBA is a right triangle //Definition of right triangle
(4) AO2=AB2+OB2 //Pythagorean Theorem
(5) AB=12 //Given
(6) r=OB=x
(7)AO=x+8
(8) (x+8)2=122+x2 //substitute for AB, OB and AO
(9) x2+16x+64=144+x2 //expand (x+8)2
(10) 16x+64=144 //Subtract x2 from both sides
(11) 16x=80 //Subtract 64 from both sides
(12) x=5=r //Divide both sides by 16, x is hte radius
