What are perpendicular lines in geometry?

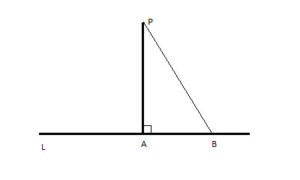
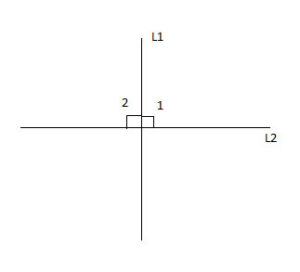
A special case of intersecting lines is when two lines intersect at an angle whose measure is 90°. Such lines are called perpendicular, and the 90° angle is called a “right angle”.

Note that in this special case, all 4 angles formed by the intersection – the opposite angels and the supplementary angles – are equal to each other, as they are all 90°.

The opposite angles are equal, as we have already proven for any pair of opposite angles, and the supplementary angles are equal because they sum to 180°, so if one is 90°, the other must be 180°-90°=90°. Right angles are typically drawn with a square, and two perpendicular lines are noted with this symbol: ⊥

We can now easily prove several theorems related to perpendicular lines and parallel lines.

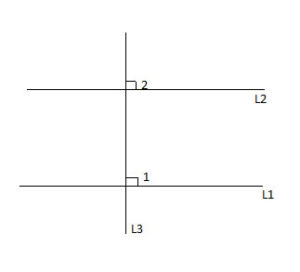
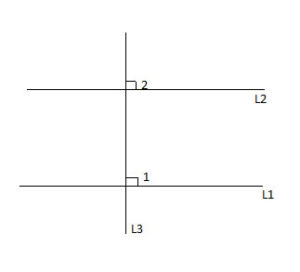
The first is called the Linear Pair Perpendicular Theorem, which states that if two straight lines intersect at a point and form a linear pair of equal angles, they are perpendicular.

This is the converse of what we stated above, that if two lines are perpendicular then the angles at their intersection point are equal.

--
Now that we've explained the basic concept of perpendicular lines in geometry, let's scroll down to work on specific geometry problems relating to this topic.