The intersecting chords theorem states that when two chords intersect at a point, P, the product of their respective partial segments is equal.
In other words: AP*PB=CP*PD
Problem
Prove that when two chords intersect in a circle, the products of the lengths of the line segments on each chord are equal.
Strategy
There are two hints given in the problem statement. The first hint is that it asks to show an equality of the product of two line segments.
This should immediately bring to mind a relationship we learned when studying similar triangles - which is that in similar triangles there is a relationship between the product of line segments- if ΔABC∼ ΔDEF, then AB*EF = BC*DE
So we will need to draw triangles where these line segments (AP, PB, CP, PD) are sides of the triangles, and then show the triangles are similar.
Let's draw it:
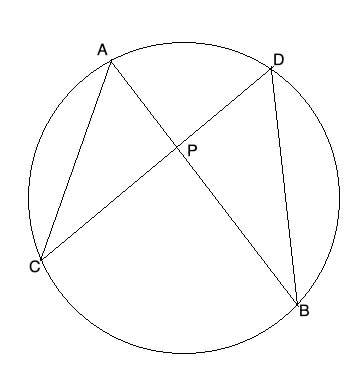
But how will we prove the triangles are similar? The second hint is that we are dealing with chords. And when we drew the lines that form the triangles (AC and DB) we created several inscribed angles that are on the same arcs. So by the inscribed angle theorem, they are equal.
We will now show that the triangles are similar by showing all their angles are equal, and we are done.
Proof
Here's how you prove the Intersecting Chords Theorem:
(1) ∠CAB≅∠BDC //inscribed angle theorem, both subtend the arc CB
(2) ∠ACD≅∠ABD //inscribed angle theorem, both subtend the arc AD
(3) ∠APC≅∠DPB //Vertical angles, or alternatively, third pair of angles in a triangle where the other two pairs are equal
(4) ΔACP ∼ ΔDBP //Angle-Angle-Angle
(5) AP*PB = DP*PC //corresponding line segments in similar triangles
