One of the basic properties of triangles is that the sum of the measure of angles, in every triangle, is 180°. We will now prove this, using what we know about parallel lines and the angles formed by a transversal line.
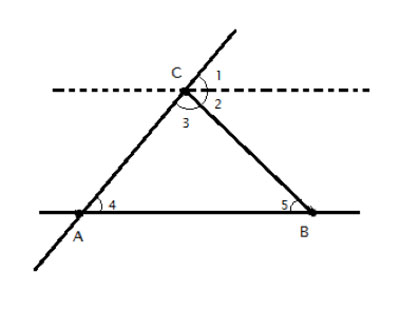
Take the triangle ABC, formed by the line segments AB, AC
How do we know we can do this? Remember the axiom we stated in the previous section: “for every straight line and every point not on that line, there is one straight line that passes through that point, and never intersects the first line.”
Here we have a straight line (AB), and a point (C ) not on that line, so we know from the axiom that we can draw a line through C which will be parallel to AB.
So we now have two parallel lines (AB and the dashed line through C). And those two parallel lines are intersected by 2 transversal lines – AC and BC, which form the triangle.
Strategy
The key to this proof is that we want to show that the sum of the angles in a triangle is 180°. And we already know that a straight line's angle measures 180°. So we look for straight lines that include the angles inside the triangle.
Proof
(1) m∠1 + m∠2 + m∠3= 180° // straight line measures 180°
(2) m∠1 = m∠4 //Corresponding angles of parallel lines, with AC as transversal
(3) m∠2 = m∠5 //Alternating interior angles of parallel lines, with BC as transversal
(4) m∠4 + m∠5+ m∠3= 180° //using (1),(2) & (3) and performing algebraic substitution. We replace m∠2 with the equivalent m∠5 and m∠1 with the equivalent m∠4
